3 years ago
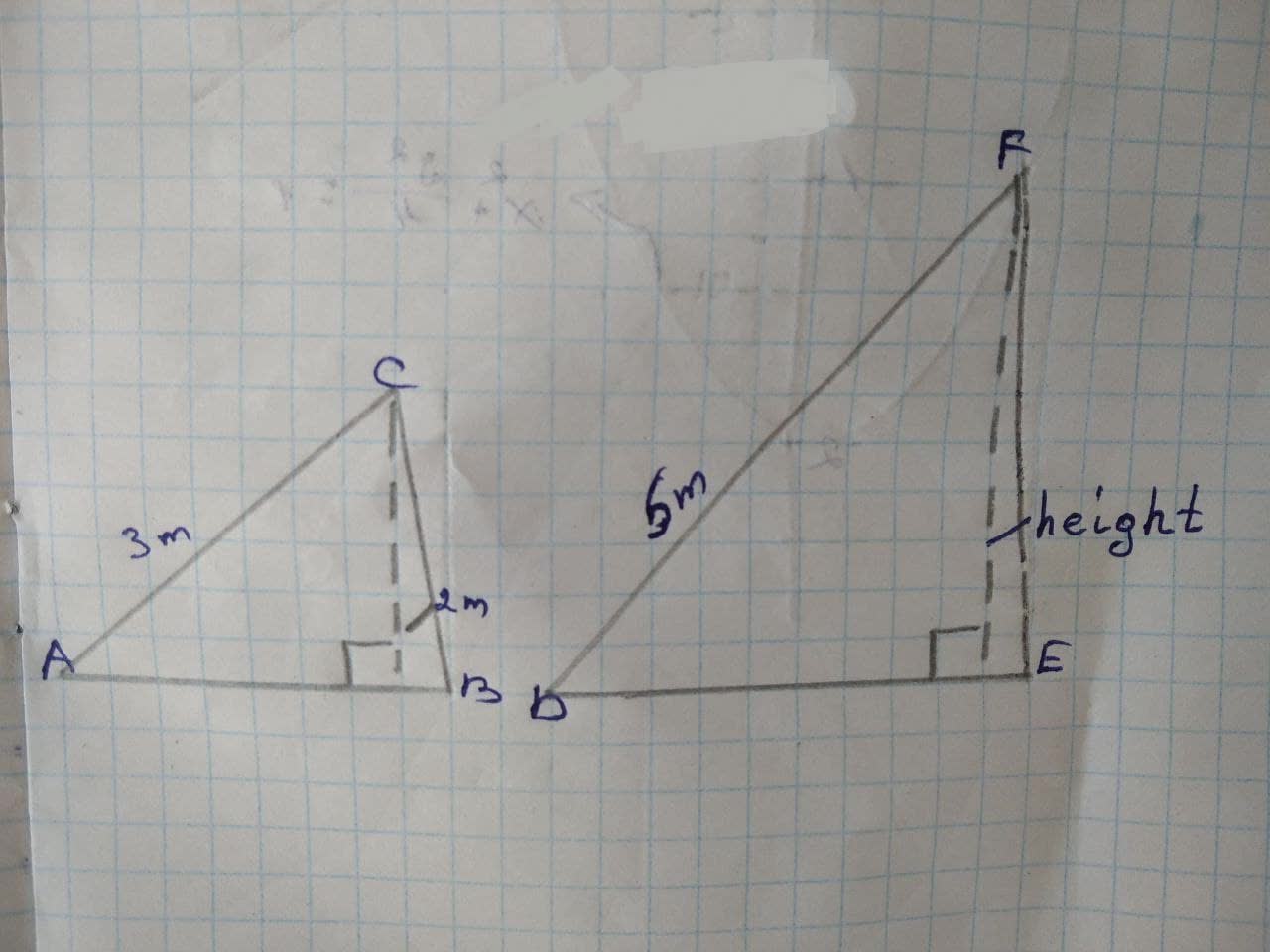
Triangles ABC and DEF are similar triangles. Solve. Round to the nearest tenth.
Find the height of triangle DEF.
underline( )m
1 Answers
Best Answer
bocchero Staff answered 3 years ago
bocchero Staff answered 3 years ago
Step 1
Here, triangu ABC and DEF are ~ilar triangles.
Need to find the height of DEF.
For the ~ilar triangles , theralios of the corresponding sidos are eguol and also the corresponding heights are egual to the corresponding sides.
Therefere, we can lorite.
(AB)/(DE)=(AC)/(DF)=(BC)/(EF)=(height of ABC)/(Height of DEF)
Substitute the values of known sides, we get
|(AB)/(DE)=(3)/(5)=(BC)/(EF)=(2)/(Height of DEF)
therefore (3)/(5)=(alpha)/(Height of DEF)
arrow Heihgt of DEF=(5* 2)/(3)
=(10)/(3)
=3* 3 m